Comparison Bars

02 May Comparison Bars

What is the math model teachers skip the most?

You guessed it, comparison bars.   Depending on the curriculum you use, you might also have heard them called tape diagrams.  They are essentially the same thing.

I get it, they seem complicated. Why can’t we just use keywords? Why? Because math models like the comparison bars help us understand problems.  Key words are tricks that often lead to number picking without thinking.

Do you hate problem-solving?  Do you your students, children, neighbors hate problem-solving?  It’s probably because keywords don’t actually work. Keywords are a pattern in math problems, but not a rule.  Relying on them is teaching our mathematicians that quick tricks will solve their problems instead of truly understanding the problem’s situation.  Sometimes how many more means to subtract, but then it could mean to add…and sometimes multiply, divide, etc. You get the point. So, this week I decided to do a mini tutorial on comparison bars, the various language they come in, and some scaffolding strategies to help you make it through!

There is so much more to be said about keywords, but this week, we will leave it at that and focus on the importance of the comparison bar.

The comparison bar is a length model.  It shows us how many more, how many less, who has more, who has less, etc.  It is NOT a break apart model such as a math mountain or number bond.

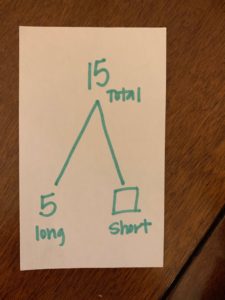
A math mountain problem would be something like,

There were 15 shirts.  5 were long sleeved the rest were short sleeved.

This is an example of a math mountain or break-apart problem.  We have a total number of shirts.  None of the shirts went away.  We didn’t get more shirts.  We just had a total and broke it into two parts (long sleeved and short sleeved).

To be a comparison problem, it would say something like,

There were 5 long sleeved shirts.  There were 10 short sleeved shirts.  How many more short sleeved shirts were there than long sleeved?

In this example, we are comparing more and less, so a comparison bar ‘shows’ us this.  Could we use a math mountain to solve? Sure, but it doesn’t help us understand the problem.  A math mountain or number bond doesn’t show us with the model’s parts someone has less, someone has more. A comparison bar does. One bar is long, one bar is short.

Check out this week’s video to help you understand how best to use comparison bars in your classrooms– kindergarten through 6th grade (&beyond!).

Do you feel empowered to use this model now?  I hope you are both empowered and inspired to use comparison bars to bring deeper understanding back into the classroom, while we take the keyword approach and throw it out with the other bag of math tricks.   What do you think? What else are students struggling with in relation to comparison bars? What else works? Comment below!