04 Mar When Wrong is Right

“Describe a square.”

“It has four sides and four corners.”

“So, like this?”

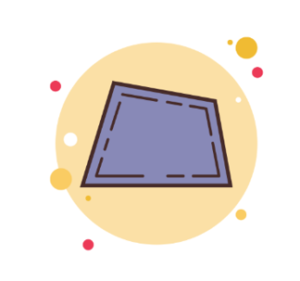
The kindergarten students scrunched up their faces in confusion.  This has four sides and four corners.

As I had kinders turn and talk about what they thought, we were able to help them clarify that a square has four equal sides, 2 sets of opposite parallel sides.  We also know they have right angles, etc. etc. but we didn’t go there with kinders. Students didn’t come up with parallel, but they did come up with four equal sides and were able to draw what they meant.

I had the kinders explain once more why the drawing I first drew was NOT a square.  I then drew a square using their more precise vocabulary.  Everyone shook their heads—yes!  That’s a square!

Next, I drew a square turned, like below.

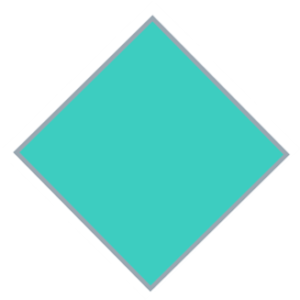
“Is this a square? “

“No!”  Every kinder was convinced it could not be a square.  As we dug deeper, they explained thought it was a ‘diamond’ (rhombus- which was also true, but more importantly, they no longer thought it was a square).  It didn’t have a flat top and bottom (the corner was at the top), etc.

We went back to our attributes.  Did it have four equal sides?  Did it have four corners?  I took a dicut square and had students turn their squares.

“Did we get a new shape, or did we take the same shape and turn it?”  We just turned it.

Minds were blown.  Mic dropped.  My work here is done.

Lol… just kidding, it was just starting as we still had to tackle cubes.  Insert facepalm, pick the mic back up….

This is a PERFECT example of how the wrong is right.  What I mean is, using the non-example in math class is one of the most powerful moves we can make in the classroom.  The quadrilateral first drawn was a non-example. It helped us clarify what we really meant to describe.  The turned square was a ‘different’ example and both tested and built our understanding of squares.

When everyone seems to have it right, I am not asking the right questions.

It would have been such an easy lesson to show students a square and to have them identify it as a square.  It was too easy.  All students were able to identify the square when presented in one way, but it was identifying the non-square, or the square in different colors, orientations, sizes, that was more of the issue. Misunderstandings surface as I dig past the recall question and into a deeper question.   When everyone is answering correctly, I know I need to ask a better question.

Students can be so deceiving.  They identify the shape as a square because I’ve only given them one type to classify.  One size, one shape, one color, one direction (orientation).  Because everyone calls it a square, does that mean they understand what a square is? More importantly, do they understand what a square is not? I’d argue no.  I’d argue without discussing non-examples, we don’t quite know the real example and why it is right.

When we use errors and non-examples in the classroom, we are increasing rigor, depth, and a fuller understanding of a concept.  If students are only ever shown the right way, the right answer, then they can easily fall into the trap of regurgitating and often struggle to apply the concept in the context of story problems, tasks, etc.   They become stuck in DOK 1, recall, and never truly understand what makes the right, right.

There are certainly more than 4 important reasons to use non-examples and errors in the math classroom, but for the sake of time, I’m going to highlight four that I find to be true time and time again.

Errors:

1. Promotes critical thinking.  When we can defend why a non-example doesn’t work, students understand the right more deeply.  The example above is a good example of this.  When students define a square, because squares are easy to us, we assume they understand squares because they have the ‘basics,’ so we quickly move on. Instead, when we see that most students know the attributes of the square and know the name, we should be prompted to ask more complex questions to reveal their misunderstandings behind the surface responses we often receive in class.  Presenting non-examples works well for this.
2. Promotes the precision of language. As in the square example, playing on what they said (even if it’s not what they meant) forces students to be more precise with their language.  Precision matters.  Explaining why something is ‘right’ doesn’t require as much precision as explaining why something is not correct.
3. Connects our intuitive ideas to correct ideas. Connecting errors (non-examples) to correct examples allow students to see what parts they have and what parts they were close to having.   When we instantly correct student’s work to make it ‘correct’ we often rob students of the opportunity to see why their answer or method wasn’t correct.  This tends to cause students to be compliant and not care about why what they didn’t work.  If we do this enough to students, I start to find that students no longer trust their intuitive math identities because when they do trust them they are corrected, but not taught.

What if instead, we honored their thinking by helping them connect their ideas?  When we show examples and non-examples, we can capitalize on moments that allow students to see what parts of the non-example work and where they don’t work.

In several recent kindergarten lessons, we worked on teen numbers.  Just as expected, when asking students to create a teen number such as 12, some students built a one and a two and some students built twelve with a ten and a 2.  I didn’t’ show the ‘correct’ answer first.  Instead, I looked for examples that were close but not quite correct or efficient.  I start with the furthest from what I want, connect to what I want and end with what I wanted.   See the figures below and the descriptions for what the student DID understand, but how we could connect and grow their idea to the next.

Fig. 1: The student was disorganized in counting and had a tough time remembering what had been counted.  14 was created with a one and a four.  We counted together (under the document camera) and allowed students to recount counters so that they would argue over the number.  This proved our point.  We then had them turn the one and the four over and counted the dots.  We got 5.  Is 14 the same as 5?  No!  So although it looks like a 1, it is really a ten.

Figure 2:  This one is closer!  This child was more organized but had to count each counter to know their total was 13.  Better—but not yet as sophisticated as we were going for.  They did use a ten and a 3 so we could turn them over and count to know it really was 13.

Figure 3:  We ended with this one.  This student made a group of ten and extra.  It was easier for us to make the ten, then count it as TEN and 3, knowing it was 13.  They also used the right purple cards.  Next, kinders were asked to revisit their own ideas at their seats and revise if needed so that they too had a group of ten and extras.

In these conversations, we can also capitalize on empathy. ” What do you think this person might have been thinking?? “Can you see why they might have been thinking that?”

Lastly…

4. Fosters a community that honors errors and can orient to each other’s ideas even if it isn’t our own idea.

As a classroom teacher, I had all the growth mindset posters.  I proclaimed daily how making mistakes was ok and how mistakes help our brain to grow!  But, yet, I spent very little time discussing the errors.  I spent time quietly correcting the errors with individual students, but what I presented to the whole class, praised, etc. was not the errors but rather the ‘right’ answers and the answers done ‘my way’.  This spoke volumes to my students about what I valued. It said, “I don’t really value your thinking, I value the right answer.  I don’t really value your mistake; I value more if it is correct.” I realized many years later that I gave lip service to errors— I didn’t actually value them.  If I truly believe errors make us grow, then I must spend time discussing them!

Take Puzzled Penguin as an example.  If you use the series Math Expressions by Houghton Mifflin Harcourt, you know that Puzzled is the mascot of that program and shows up in every unit, for every grade.  He tends to present a scenario in which he is puzzled and needs the students to help him.  We can easily overlook this opportunity and in fact most teachers I know gloss over this part of the lesson when in fact it can be the most meaningful part of the lesson.

In a recent 4^th grade classroom, we were learning about comparing fractions that had common numerators or that had common denominators.  Puzzled appears in the lesson a bit confused.  He begins to explain how one night he ate of the fish and the next night he ate of the fish.  Because 6 is greater than 4, he ate more the first night.

Students instantly want to correct Puzzled.  Why wouldn’t they?  Every time they get something incorrect, they are instantly corrected.  Instead, I take the opportunity to support empathy.

“I totally understand why Puzzled thinks this way.  Can you explain to a neighbor why Puzzled’s idea makes sense and where his misunderstanding lies? “Students discuss, but now empathizing with Puzzled,

“I totally see why he would think that.  It is weird that in whole numbers 6 is greater than 4, but in fractions, that’s not still true.”

T: “Let’s build on that idea.  Instead of telling Puzzled what error he has made, come up with three questions you could ask Puzzled to find his own error.”

Students came up with the best questions.  Questions like:

• What do you remember about what the denominator means?
• Did you use your fraction bars to prove your thinking?
• How are fractions different from whole numbers?

These were just a few of their examples.  Now, instead of laughing at him for making another error, or quickly identifying an error and moving on, we capitalize on empathy, understand more fully why the error is significant, and have now up-ed the DOK level from a 1 or 2 to a 3 or 4.

When I present work to the class, I often look for ideas that aren’t quite there so that I can honor the thinking that IS there but then use the errors to build a deeper understanding of what is correct.

Another example happened just this week in a classroom in which we were discussing time.  Michelle, a fabulous 3rd-grade teacher asked students to write down the time (as seen on her clock).  One student wrote the time on the whiteboard.  We can quickly correct the student (privately), but when most of the students had the correct time, I think:”I need to challenge their thinking.” Presenting the error will help both the spectrums of students.

“Discuss with your partners how a mathematician could think this was the correct time. Don’t discuss the right answer, discuss how or why this mathematician thought THIS answer.”  (Do you see it? The student mixed up the hour and minute hands, they wrote 7:15 instead of 2:35.)

This question was much tougher but really forced students to think deeply about this common error.

Understanding the incorrect helps us to more deeply understand the correct, this makes the ‘wrong’ answer the ‘right’ example to explain and to teach from.